- Maria Blanton
- https://www.terc.edu/display/Staff/Maria+Blanton
- Senior Scientist
- Presenter’s NSFRESOURCECENTERS
- TERC
- Angela Gardiner
- https://www.terc.edu/profiles/angela-murphy-gardiner/
- Senior Research and Design Specialist
- Presenter’s NSFRESOURCECENTERS
- TERC
- Ana Stephens
- https://wcer.wisc.edu/About/Staff/1213
- Researcher
- Presenter’s NSFRESOURCECENTERS
- University of Wisconsin Madison
Project LEAP: Extending a Grades 3-5 Early Algebra Learning Progression into ...
NSF Awards: 1720129, R305A170378
2022 (see original presentation & discussion)
Grades K-6
This video will showcase two related research projects focused on designing an effective and inclusive Grades K–2 early algebra intervention. Our design was based on our Grades 3–5 early algebra intervention, which has been shown to significantly improve students’ algebra readiness for middle grades across all demographics. In this video, we highlight features of the intervention and describe ways we have observed young learners thinking algebraically as they progress through it. This intervention offers an important curricular resource for teachers in building classrooms that foster children's algebraic thinking.
Related Content for Broadening Access to Algebra: An Intervention for Grades K–2
-
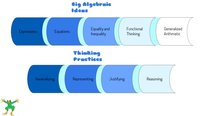 2018Impact of a Teacher-Led Early Algebra Intervention
2018Impact of a Teacher-Led Early Algebra Intervention
Maria Blanton
-
 2018MathByExample: Dislodging Misconceptions in Grades 4/5
2018MathByExample: Dislodging Misconceptions in Grades 4/5
Allie Huyghe
-
 2019Behind the Development of MathByExample
2019Behind the Development of MathByExample
Allie Huyghe
-
 2022Designing Asset Based Math Interventions with Serious Games
2022Designing Asset Based Math Interventions with Serious Games
Jessica Hunt
-
 2020Pirate Math Equation Quest
2020Pirate Math Equation Quest
Sarah Powell
-
 2017Conceptual Model-based Problem Solving: A RtI Program
2017Conceptual Model-based Problem Solving: A RtI Program
Yan Ping Xin
-
 2017Young Children's (K-1) Understanding of Algebraic Thinking
2017Young Children's (K-1) Understanding of Algebraic Thinking
Bárbara Brizuela
-
 2017Preparing Student for Success in Algebra
2017Preparing Student for Success in Algebra
Maria Blanton
Past Showcases
Opinions expressed on this site are those of the contributors and not necessarily those of the National Science Foundation.
















Maria Blanton
Senior Scientist
From the Project LEAP team, thank you for taking a moment to view our video! We are excited to share this final stage of our work designing an effective early algebra intervention for elementary grades. We look forward to answering your questions during this Video Showcase. We would be glad to share more about our intervention, how it improves students’ algebra readiness, and how you can get the LEAP curriculum in your schools. Something to think about:
What are your experiences with early algebraic thinking?
If you are a secondary mathematics educator, what are some of the challenges your students face that might be addressed in the earlier grades?
Alejandra Duarte
Cassandra Hatfield
This is really exciting work and related to our numeric relational reasoning formative assessments! Supporting teachers with instructional decisions and then intervening with high quality lessons to support algebraic thinking are so critically important! Exciting work!
Angela Gardiner
Senior Research and Design Specialist
Thanks, Cassandra.
Eva Thanheiser
Ana great video ... thanks for sharing your work and checking out ours. I would love for us to connect about how to leverage each others' work.
Ana Stephens
Researcher
Thanks, Eva! Yes, would love to connect! One challenge I see is that real-world data/issues are often messy whereas when our young students first encounter (for example) functions, their "data" are perfectly linear (or quadratic, or exponential). But seeing math as a tool to describe the real world is so important.
David Kung
Director of Policy
Love seeing how algebraic thinking is appropriate - even vital - in early grades!
The attention to equity is admirable – but it only got a brief mention in the video. How are the lessons and interactions structured to address EDI issues?
Absent longitudinal data, what does evidence look like to suggest these students will fair better when they get to later, middle- or high-school Algebra I classes?
The picture of balances make me wonder if kids are also building early physics knowledge. Is there anything in the literature about concepts of levers and torque in such early learners?
Angela Gardiner
Senior Research and Design Specialist
Thanks for your questions David, and thanks for watching.
How are the lessons and interactions structured to address EDI issues?
We worked with different advisors to develop a curriculum that was culturally and developmentally appropriate. We tested this curriculum in several settings both in schools with low SES status and in schools with high percentages of students with learning differences. We incorporate discussion and manipulatives in all our lessons, which we have found help students who have learning difficulty in math. We have included a section in our curriculum which provides strategies for teachers to support struggling learners. This was developed with the help of several advisors who have extensive research experience on students with learning differences, as well as from teachers who work with a variety of students every day in their classrooms.
Absent longitudinal data, what does evidence look like to suggest these students will fair better when they get to later, middle- or high-school Algebra I classes?
In a large-scale, randomized study we found that students who took our Grades 3 – 5 intervention as part of their regular instruction were significantly more prepared for algebra than those who had only their regular curriculum. These findings held for students from schools with 100% low SES status and 90+% students of color.
The picture of balances make me wonder if kids are also building early physics knowledge. Is there anything in the literature about concepts of levers and torque in such early learners?
We haven't researched this area, but I can see the connection as well. It would be interesting to look into.
Myriam Steinback
Consultant
How great that you are able to bring LEAP to K-2! Young students are able to have algebraic ideas - if we get away from the 'x and y' mindset - and your design shows that. I wonder about the 18 lessons - how and when are they designed to be used? What support, if any, do teachers need to facilitate the lessons?
Angela Gardiner
Senior Research and Design Specialist
Thanks for watching, Myriam. This is such a fun age group to work with. The 18 lessons are used throughout the school year, teachers teach approximately 1 lesson a week and each lesson ranges from 30 mins to 1 hour depending on grade level (K-2 is 30 mins a lesson). Teachers we have worked with have found this very doable with their regular math curriculum. Professional development is available, and we do recommend it. We have worked hard, however, to make sure the lesson are easy for teachers to follow and understand because we realize not all districts have time or can afford PD. In the future we would like to incorporate a suite of videos that would be accessible to teachers who can't participate in the PD, but would like a little more information before beginning a lesson in their classroom.
Myriam Steinback
Meixia Ding
Thank you for sharing this exciting project! It is very encouraging and impressive to see the figure that shows the performance difference between the intervention and the control groups! I also have a question about the intervention (18 lessons). It looks like these 18 lessons were added as extra materials to the regular math curriculum for the intervention group, right? How did you deal with the factor of content exposure? In other words, has the intervention group received 18 more lessons than the control group? Did I have a misunderstanding here?
Leanne Ketterlin Geller
Such interesting work! Connecting algebra learning to the K-2 classroom is so important. We are developing classroom assessment resources that tie in some of these early numeracy concepts that focus specifically on numeric relational reasoning. I see a lot of useful cross-overs with your intervention. So excited to see where this work goes. Thanks for sharing!
Angela Gardiner
Senior Research and Design Specialist
Thanks Leanne. I am familiar with your project, and viewed your video earlier today, job well done!. I also see a connection, maybe our paths will cross in the future. We have been tinkering with the beginnings of an EA digital assessment tool, we may need connect down the road. Thanks again for checking our work out!
Ana Stephens
Noelani Ogasawara Morris
Demonstration Teacher
I completely agree that introducing the concepts of algebraic thinking and developing relational thinking across the equal sign is so valuable at the early elementary grades. I notice that the LEAP program uses a variety of tools and what appears to be guided strategies for problem solving. Is there room and flexibility for students to use their own strategies to problem solve and share their thinking to their classmates?
Angela Gardiner
Senior Research and Design Specialist
Hi Noelani,
Great question! The answer is YES!, we encourage a wide range of discourse and encourage children to share their math thinking and strategies in every lesson. We discuss with students, on a regular basis, that there are often multiple ways to solve a problem, and we share as many as possible. LEAP discussion is also student driven, so often students present the ideas or solutions and discuss with each other in whole group meetings. I believe the algebraic thinking concepts are important, but math discussion in elementary classrooms is also VERY important, and often doesn't happen enough. We try to highlight the importance of math discussion throughout the curriculum and when we work with teachers.
Noelani Ogasawara Morris
Demonstration Teacher
Wonderful- I love hearing about educators who value, if not more, the process of problem solving versus the final answer. While efficiency and accuracy are definitely important, building that number sense and flexible thinking in the early years are so valuable in their lifetime as mathematicians!
Angela Gardiner
Janet Stramel
I LOVE that you are teaching algebra with the elementary students. I have found that some people (even children) are afraid of the word "algebra." Do you tell them they are doing algebra and just doing the math?
Angela Gardiner
Senior Research and Design Specialist
Hi Janet,
I agree that people are afraid of the word algebra. When we did our grades 3-5 intervention we spoke with students about how the math they were doing was the beginning stages of algebra, and the children LOVED it! They would go home and tell their parents they were doing algebra and work with older siblings on algebra homework, they thought it was a BIG deal. We don't mention it at the K-2 level as much, and really the work we are doing with these students transcends all types of math. We encourage them to use the strategies we teach them in LEAP lessons in all their math work, we don't want the focus to be "we do this type of math when we do LEAP or algebra". Thanks for taking and interest in the video, and for this great question.
jennifer Knudsen
Great project and video! When you show what the K-2 students can do, it's amazing! What was it like to move down to that grade level from g 3-5?
Ana Stephens
Researcher
Thank you, Jennifer! One change was that we used more hands-on activities in the younger grades, including the use of balance scales to model mathematical equivalence and Unifix cubes to model even and odd relationships and arithmetic properties. We also see perhaps even more connections to traditional arithmetic goals at this age. For example, as students discover and discuss the Commutative Property of Addition, they are simultaneously working on arithmetic skills. They later put these properties of operation to use to compute more efficiently (e.g., when thinking about how to compute something like 9 + 5 - 5).
Cynthia Orona
Thanks for sharing! I totally agree with the need to teach algebra in the early, formative years.
Ana Stephens
Researcher
Thanks for visiting, Cynthia! I just watched your video and enjoyed it as well!
Peter Tierney-Fife
Thanks so much for sharing this work—it's very important and exciting! I am wondering if you can share more about the student-driven discussions and the approaches or resources you use to help students build their communication skills and support their discussions.
Your approach seems to include rich problems/activities with a lot to talk related to them, which is great. I'm wondering more about particular language strategies or discussion aids, and most specifically, I'd love to know if you have particularly effective or favorite approaches to support students who are multilingual learners in English language mathematics classrooms.
Thanks in advance!
Ana Stephens
Researcher
Thank you for this important question. We don't have all the answers, and I hope we can learn from others here (I just watched your video and love the focus on students' mathematical strengths--it makes me think about broadening the definition of "good at math.") I will share that we think our focus on visual representations helps all students, including multilingual learners. We model mathematical equivalence with balance scales. We use visual representations of even and odd numbers using dot cards (seen in the opening scene of the video) and Unifix cubes. We also model arithmetic properties (e.g., the Commutative Property as seen in the video) with physical materials.
We do also focus on particular vocabulary. For example, as early as Kindergarten we discuss with students the meaning of "conjecture" and "argument" and revisit these meanings throughout the lessons as we ask them to make and test conjectures. We talk about even and odd numbers in terms of "pairs" and "leftovers" and spend some time "acting out" these ideas. For example, students might be asked to figure out if there is an even or odd number of students in the class without counting. We also talk explicitly about the meaning of the equal sign--often replacing the equal sign with "is the same as" when we read equations--and use gesture when talking about "balance" in equations and the "sides" of equations.
Peter Tierney-Fife
This is helpful, thank you. I share your thinking that use of visual representations helps all students, including students who are multilingual. My work includes thinking about ways visual representations (right now, mostly diagrams like single or double number lines and tape diagrams) and receptive and productive language strategies can be combined in activities for mathematics teacher professional learning, and I appreciate your information on combining visuals with gestures, acting out, and careful vocabulary work with students in LEAP. Thanks again.
Ana Stephens
Maria Blanton
Senior Scientist
Thank you Peter. Visual and concrete representations (and gestures!) have been key to helping young children in our study understand algebraic ideas. It's fascinating how particular tools can challenge their thinking about "simple" concepts like the equal sign and help them begin to think algebraically. I'm curious about double number lines and tape diagrams and how you use these. Do you have a reference you can share? I thoroughly enjoyed your video. One of the delights of our work - which I appreciated in your video as well - is talking to young learners and listening to their thinking and ideas. They always have wonderful insights to share!
Peter Tierney-Fife
Maria, I agree it is a delight to talk with young learners, and I think a responsibility to listen well to their thinking and ideas. The most available resources I can share are from a different project, Visual Access to Mathematics (if interested, see our old video on the multiplex which has some context and resources in comments). It's focused on grades 6–8, ratio and proportional reasoning. We also have some example activities online and, for use of double number lines in percentage contexts, there are more resources associated with this collection of percentage change apps. One of my favorite sets of resources makes connections between double number lines and the coordinate plane and includes this Double Number Line to Coordinate Graph app.
Maria Blanton
Senior Scientist
Great - thanks for the resources Peter!
Zach Mbasu
When kids understand Algebra other challenges go down! This is an amazing Algebra intervention and I would definitely want to learn more. In my country there are challenges that remain, particularly in the area of equity and equality. I am wondering how can I provide this kind of learning to girls and boys in remote, rural, and hard-to-reach areas in Africa that have no access to teachers?
Maria Blanton
Senior Scientist
Hi Zach - thank you for stopping by! We totally agree that algebra is so central to mathematical success! Feel free to email us for more information about the intervention. We'd be happy to talk further. We have a companion intervention for Grades 3 - 5 which we developed prior to our K - 2 work. We found that students from marginalized communities who had this intervention as part of their regular curriculum significantly outperformed peers in similar demographic settings who had only their regular curriculum. So we know this approach is effective for all students! You describe a difficult challenge, but we would be happy to talk more. Also, I wanted to say I loved your video. It is truly amazing how students around the world can connect and learn with and from each other!
Carol Lumm
Congratulations Maria, Angela and the rest of the LEAP team. Very informative video and I'm enjoying following the discussion of your work. Thanks for your contributions to the important topic of introducing young students to algebraic thinking.
Maria Blanton
Senior Scientist
Thanks so much Carol!
Abigail Helsinger
This is great! I love hearing about these early introductions to algebra. I use algebra everyday in the "real" world. It's so important kids learn the skills early and then understand how critical the skills are in everyday life. Thanks for your work!
Maria Blanton
Senior Scientist
Thanks so much Abigail! We agree that this is so important for young learners. Thanks for stopping by!
Further posting is closed as the event has ended.