- Peter Tierney-Fife
- Curriculum/Instructional Design Associate
- Presenter’s NSFRESOURCECENTERS
- Education Development Center (EDC)
- Pamela Buffington
- http://ltd.edc.org/people/pam-buffington
- Co-Director
- Presenter’s NSFRESOURCECENTERS
- Education Development Center (EDC)
- Josephine Louie
- Senior Research Scientist
- Presenter’s NSFRESOURCECENTERS
- Education Development Center (EDC)
- Jill Neumayer DePiper
- Senior Research Associate
- Presenter’s NSFRESOURCECENTERS
- Education Development Center (EDC)
- Johannah Nikula
- Senior Project Director
- Presenter’s NSFRESOURCECENTERS
- Education Development Center (EDC)
Visual Access to Mathematics: Professional Development for Teachers of Englis...
NSF Awards: 1503057
2019 (see original presentation & discussion)
Grades 6-8
Mathematics educators participating in the Visual Access to Mathematics professional development learn to use visual representations and language strategies to support their grades 6-8 students who are English learners (ELs). The hybrid online and face-to-face course was developed through an iterative process by a collaborative team of practicing educators, PD developers, and researchers. The course focuses on the combined use of visual representations and language access and language production strategies in ratio and proportion problem-solving lessons. Educators explore their own use of visual representations and also analyze both paper-and-pencil and video work done by students who are ELs. The video includes initial findings from the cluster randomized control trial during the 2017-2018 school year.
Related Content for Designing PD for Math Educators of Students who are ELs
-
 2016BREAKING DOWN LANGUAGE BARRIERS: MATH FOR ELLS
2016BREAKING DOWN LANGUAGE BARRIERS: MATH FOR ELLS
Brittany Webre
-
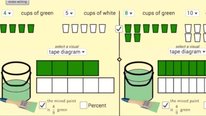 2016Visual Access to Mathematics: Supporting Teachers of ELs
2016Visual Access to Mathematics: Supporting Teachers of ELs
Johannah Nikula
-
 2016An Early Childhood STEM Professional Development Model
2016An Early Childhood STEM Professional Development Model
Alissa Lange
-
 2017All Included in Mathematics - Expansion and Dissemination
2017All Included in Mathematics - Expansion and Dissemination
Daniel Heck
-
 2019MC² Summer MathLab
2019MC² Summer MathLab
Wanda Bulger-Tamez
-
 2019STEM for English Language Learners with Lehman College-NWHS
2019STEM for English Language Learners with Lehman College-NWHS
Serigne Gningue
-
 2019Video in the Middle Online Mathematics PD
2019Video in the Middle Online Mathematics PD
Nanette Seago
-
 2020STEAM Your Way 2 College
2020STEAM Your Way 2 College
Shakhnoza Kayumova
Past Showcases
Opinions expressed on this site are those of the contributors and not necessarily those of the National Science Foundation.


















Peter Tierney-Fife
Curriculum/Instructional Design Associate
Thank you for watching our video. We are currently conducting analyses during the final year of our project, and we are preparing to disseminate this work and our findings more broadly. We are interested in learning what information would be most useful for various audiences to know about this project and its associated research. What would you like to know about the project and research, or what do you think people in other roles might find most interesting? We look forward to your feedback and questions.
Catherine McCulloch
Hi Peter, great video. You mention that you are using apps in this project. Would you say more about which apps you are using and how you are using them?
Fatai Bakare
Peter Tierney-Fife
Curriculum/Instructional Design Associate
Hi Catherine,
Thank you for your questions. We developed many apps specifically for this project that are authored in GeoGebra, so they work on most devices and they are free for everyone. The apps are on the GeoGebra Web site as a book/collection at https://go.edc.org/vam-apps, and you can also find these and related individual apps by searching for 'edc vam maine' (they are posted under the user "EDC in Maine") in the classroom resources search bar on the GeoGebra site.
The apps support understanding and working with visual representations of ratios and proportional relationships. Below are three examples of how they are used in the course (note that depending on their use, most of the examples given could be listed under more than one category):
The apps are integrated throughout the professional development in both face-to-face workshops and online sessions. Some of the apps are associated with a course activity such as a specific math task, but almost all the apps can be used with a wide variety of contexts. Some of the apps are included in the course as optional resources. Although the primary audience for the apps is educators/course participants, they can certainly be used by students and many participants report using them with students or planning to use them with students in the future.
An example way that an app is used in the course is to connect participants' knowledge of ratio and their knowledge of slope. Participants work on a task that asks them to answer questions about two given visual representations—a double number line and a coordinate graph—for the same problem context. Afterward, they watch a screencast (video) that models working with an app on the same problem context, and then they explore the app themselves. In the Double Number Line to Coordinate Graph app, dragging a blue handle on the right side of the top number line transforms a double number line to a coordinate graph.
Multiple participants have reported that using this app has been important for their own learning. Three example responses from participants are below:
"When the double number line unfolded into a quadrant of a graph with points already plotted I was struck by what a powerful visualization it was for me. It was like I was thrown into the deep-end of the algebra pool and learned a new appreciation for the graphic representation of a problem."
"When we used the applet that asked us to pull up the top line to make a set of coordinate axes I was blown away. When I taught slope to students, I didn't teach it with the current understanding of its connection to proportional relationships and similar triangles. This course has added a lot to my understanding of the connections between these representations. Thanks for pushing me outside my comfort zone."
"When I saw the applet go from the double number line to the x and y axis it was an unbelievable experience. I understood the connection of the double number line and the importance of teaching it."
We are excited to share the apps and we hope they are helpful for educators and students.
Fatai Bakare
Susan Jo Russell
Principal scientist
Hi Peter,
Very interesting video about the interaction between mathematics learning and language learning. Can you say more about how teachers integrated learning language in context with using visual representations? Are there one or two examples of this you could share that would show how teachers' classroom approaches incorporated what they learned in the course?
Thanks so much.
Peter Tierney-Fife
Curriculum/Instructional Design Associate
Susan Jo,
I appreciate the focus of your question—integrating learning language in context with using visual representations. Including the use of visual representations is such an important feature of our work. Although most of the language strategies teachers learn about and implement can be used effectively in many content areas, within the VAM course these strategies are regularly and deeply integrated with the use of structural visual representations (such as tape diagrams and double number lines), which can themselves be used to support students who are English learners as they learn language while doing mathematics.
For example, teachers design a task with a word problem that uses a partial (or ‘starter’) visual representation and sentence starters. During this process, teachers consider questions such as, “How will the partial visual representation support students’ understanding of the information in the problem?” and “What sentence starter can draw students’ attention to details in the visual representation or task?” One possible reason to use a partial visual is that it can act as a language access strategy, since its structure and features can support student understanding of the language and the meaning of the associated word problem. The use of a sentence starter can explicitly prompt this use of a partial visual.
Another example is when teachers plan a lesson that incorporates their learning about differentiating teacher questions based on the English proficiency level(s) of their focus students. Teachers wrote questions that refer to visual representations, such as, “What does the 10 [on the visual] represent in the problem?” and “[pointing to answer] show me the answer here [pointing to double number line]”.
Teachers also used visual representations as a starting point for whole-class discussions. One teacher reflected on how her practice changed during the VAM course, writing that, “I now [at the end of the VAM course] plan lessons to have time to share visuals, particularly on the document camera…. By having students look at and examine the visual representations that their peers have made, it gives them new options for their own work, and it increases math conversation and discourse between students. Visual representations also help me to help students, because they might be able to show their reasoning with a visual even though the calculations are hard or explaining their logic might be a challenge.”
Over time, as teachers implement lessons with combinations of language strategies and visual representations, and as they attend to their students’ math and language understandings during these lessons, the potential of visual representations as a support for learning language while doing math may become more salient for them. I feel the teacher reflections below capture this well:
“Previously, I hadn't considered how visual representations could help support students in language access and language production. I used visual representations in lessons because I knew they were valuable tools for solving and understanding problems, but I hadn't considered their other benefits. I now consider the other structures I can add to the use of visuals to support EL students. The videos [in the course] we watched of students really helped to solidify this understanding for me. I saw how students could use their double number line or other visual representation as a place of reference when describing their work and that the [visual] model helped ground the language of the problem in a visual structure.”
"My most important learning in the course so far is is how you can see a students thinking by using visual representations. Before this course I just thought of visual representations as a strategy to help students solve a problem but not as a way I could understand more about their mathematical thinking. I have also learned that they can use visual representations to decode the meaning of text."
"I have also adjusted how I run class-wide discussions. I now ground so many of these discussions in student work, pushing students to be specific and talk explicitly about a model. I show a student's work on the Doc Cam and have another student go up and point to what they see represented. It has made our discussions much richer and I think made my students' understanding deeper. It has also helped with discourse, as students are a tool right in front of them to comment on."
"Providing them with a visual representation of the relationship between two quantities in a double number line and in a tape diagram has helped provide something tangible for my students and I to ground our conversations in and to literally point to relationships that exist within a word problem."
Susan Jo Russell
Principal scientist
Thanks for this thorough response--very helpful! We are also using visual representations as a central tool in helping teachers incorporate mathematical argument into core math content at the elementary level. By its very nature, mathematical argument involves articulation of math ideas, and, for young students, representations are the tools for argument, so this is a natural connection. I appreciate hearing about how this work supports EL students.
Peter Tierney-Fife
Curriculum/Instructional Design Associate
I am very interested in learning any insights or findings you can share from your work around argumentation with elementary educators and students. There are so many exciting aspects to pursue and consider related to visual representations in mathematics; using visual representations can support language learning, can be a tool for argumentation and communication more broadly, can be a tool for problem-solving and mathematical modeling, can be a tool for investigating mathematical concepts, can support engagement with mathematical practices, etc. I am especially interested in continuing to learn about how dynamic visual representations, like our GeoGebra apps, are related to these purposes, especially student language learning and argumentation. Thanks again for your question.
Kathe Kanim
Your video and thorough responses suggest you have a very successful program. I am curious about disseminating the work and findings. Are the blended courses something that you will offer again? Is the course the work that will be disseminated? Would you consider your project as scalable? Often grant funded programs end with funding even with documentation of success.
Peter Tierney-Fife
Curriculum/Instructional Design Associate
Kathe, great questions.
We are working on additional analyses of the study educators' notebook responses, surveys, and other instruments that will help understand this work and its potential impacts more fully.
As we begin to disseminate more broadly, we are very interested in learning what information would be most useful for various audiences to know about this project and its associated research. Do you have ideas about this? We have multiple papers and opportunities for dissemination planned related to educator self-efficacy and other topics, but we are interested in what others would like to learn.
We are hoping to not be one of those programs you describe ("end with funding even with documentation of success"). We do consider our course and elements of it scalable, and we are actively planning additional proposals and working to determine if other methods of offering the course or related professional development are possible in the near future. We have received some feedback that collections of materials may be of interest, for example for higher education faculty for use in mathematics methods courses or other courses and programs. We have also received requests to run this course or related professional development with other mathematics topics (beyond our focus on ratio and proportional reasoning) and other grade spans, including upper elementary grades. Any suggestions or ideas related to expanding and scaling this type of work are appreciated!
Thanks
Beth Sappe
Director - STEM Mathematics
Hello,
Thanks for sharing your interesting video. We have a large EL population in my school district and are always looking for ways to better support teachers and our students. Is this a full curriculum or activities for teachers to faciliate at specific times throughout the year. I believe these are best practices for all students and wonder what the shift in student concrete understanding could look like if when engaging in visual activities throughout middle school. Do you plan to share the content developed for teacher professional learning as an open source resource?
Thanks, Beth
Peter Tierney-Fife
Curriculum/Instructional Design Associate
Beth,
Great questions!
The VAM course is blended professional development for educators and not a curriculum for use with middle grades students—in fact, it's designed so that an educator can take their learning about the strategies and representations and integrate them into any curriculum or program, or even in small group work and intervention work with students. We have also had participants who are coaches of other educators and integrated their learning in their coaching work. As previously enacted, the course includes an expectation that a participating educator picks 2 focus students to follow through the course and for whom they plan most consciously; over time they implement a variety of strategies and tasks with at least their focus students (although often educators report using the strategies with all their students, and yes, many participants have reported to us that they find these strategies help all their students). There is a fair amount of latitude and choice in these implementation cycles, including some choice related to tasks and strategies and whether and how to adapt the materials for their unique focus students, context, enacted curriculum and program, etc. Part of what we work on is related to attending to student strengths and understandings and using the information to inform instructional planning. However, we do have particular foci and parameters around each implementation "assignment".
For example, at the end of the initial VAM summer institute, educators write a plan for an activity or lesson to implement within the first six weeks of school. Their planning process includes writing at least 1 mathematics [non-language] objective and 1 language development objective, choosing a mathematics task that includes having students create, use, or interpret a visual representation, and anticipating what students at different English language development levels might do, say, and find challenging during the activity. In addition, educators must also plan to use the 3 Reads strategy (you can also read about this and other language strategies—please note this was written for a different project) in the first 6 weeks of school, either independently or integrated with the previously mentioned plan. With both expectations, educators can choose the mathematics task (from their own curriculum or from the many we've already introduced in the VAM summer institute), the timing (within the 6 weeks), the setting, and students who will do the activity, among other things.
I also wonder what a shift in student understanding could look like if students engaged in a regular and cohesive way with visual activities throughout middle school (especially if it followed directly on similar prior engagement!). In this vein, we have had course participants ask us to offer the course again so their colleagues can participate and work toward this vision, as well as ask if/when we will develop a course for elementary grades for their colleagues who teach younger students.... we are excited and motivated based on their interest, but we have no current funding to do so.
As far as sharing content as an open resource, we are currently exploring options and considering best approaches within the parameters of our current funding, which does not include extensive development work to share everything in that way with the necessary supporting materials. Also, as part of our learning, we are finding at least anecdotally through participant (educator) writing and interviews that it's important for educators to engage with the course in a sustained way with knowledgeable facilitators, so we are considering this feedback when we think about how best to share in a thoughtful and impactful way. In the mean time, we are sharing many aspects of the course and many of our materials when we have the opportunity, including during this showcase, and we are very interested in what you and others find most interesting and would recommend sharing for various audiences. I appreciate your question and please note that sharing widely is a high priority for us, and part of the reason why we developed the interactive apps for the course using the free open source application GeoGebra under their non-commercial license, which allows worldwide access and the ability to remix/adapt the apps for different languages and in any other way.
Denise Schultz
Instructional Math Coach
Hi Peter. Thanks for sharing this video and your thoughtful responses to our questions. Your project is addressing a huge area of need in education, especially in the U.S. As a facilitator of professional learning around teaching mathematics (in my own district) I tend to suggest the use of visual representations as a go to when working with teachers of english language learners but find that teachers are often limited in their access to visual mathematics depending on the curricular materials teachers have available to them. Pulling together and producing the right visual image for their students is time consuming for teachers so I think your project of supporting teachers' access to visual mathematics is key to improving our instructional practices. I am especially interested in the apps and the visual images that can be manipulated and love the idea of creating a toolbox of interactive math visuals for our teachers of ESL/ELL students. Does your project include access to interactive math visuals or can you recommend free resources for our teachers to use?
Peter Tierney-Fife
Curriculum/Instructional Design Associate
Denise,
My experience mirrors your comment that some middle level mathematics educators lean away from using visual representations with grades 6-8 students, and the reasons vary but include access to what they consider appropriate tools as well as their own level of comfort and familiarity with using visual representations as tools for problem solving or for other purposes.
Although we encourage participating educators to explore and use any type of appropriate mathematical visual representation, most of the VAM materials focus on tape diagrams, double number lines, and coordinate graphs. We emphasize connections and differences between them, including affordances and limitations of them relative to each other and the contexts of mathematics tasks.
This set of key visual representations share some characteristics; we may at some point articulate and share these characteristics more thoughtfully, but I'm giving you my personal off-the-cuff response on this for now, in the hope that it's helpful. For example, we believe these visual representations can support students who are English learners gain proficiency with the grades 6-8 Common Core mathematics standards and the Standards for Mathematical Practice; they can be useful as tools during ratio and proportional relationship problem solving, including for representing ratio relationships structurally and supporting decontextualization and re-contextualization (yes, these characteristics overlap considerably); they can be used with and as supports for language development; they are already in relatively common usage in many elementary and middle grades curriculums and materials (even double number lines are more widespread now, which I am pleased to see); they can be used successfully with pencil and paper and educators can integrate them fairly easily in materials they create themselves (toward this end, one of our apps is designed for educators to make their own double number lines for their own tasks); as a set of representations they support a coherent progression and the ability to make deep connections across grades 6 to 8 concepts related to ratio and proportional relationships, including geometric similarity and slope.
Of course, limiting the number of types of visual representations also supports educators gaining comfort and familiarity with those specific representations, which may support educators using them more frequently, flexibly, and/or for different purposes with students. As part of this process, we ask educators to analyze student work with visual representations (we use screencasts of sample work as well as require them to analyze the work of their own focus students) with an emphasis on noticing student strengths and wondering about student thinking.
The free interactive apps developed for the VAM course (for more information about them see my responses above) also focus on these 3 types of visual representations, but some of the apps include other types of representations as well (area models, set models, etc.). These apps are designed with middle level educators and students in mind, and have been revised with middle level educator feedback over time, so they may appear different from some other apps you see online.
In addition, we have created some Desmos card sort activities that we use as part of our online sessions and that we discuss during some of our videoconference meetings (you can see this example card sort in the video around 1:53).
You can check out other GeoGebra resources and apps on other sites, including the PhET math simulations and their related video about the PhET math sims that is part of this showcase.
I encourage you to continue supporting the use of visual representations when working with mathematics educators of students who are English learners, and I hope you find our resources helpful. In this spirit of encouragement, I'm including some example quotes from VAM course participants:
"I have always struggled with visual representations in math and have found over the course of this [VAM] class, it has become easier to not only use them to solve problems, but also teach my students how to use them to solve and EXPLAIN problems." [capitalization was in the original educator response]
"The visual representations presented in this [VAM] course have ALL been completely applicable and implementable immediately in my classroom. The power of the visual representation to lessen the language-load is significant and as the number of ELs that I teach continues to increase, the more important the visual representations will become." [capitalization was in the original educator response]
"Prior to this course I had thought of visual representations as presentation tools, ways of showing and explaining my work after I had solved the problems. Using VRs [visual representations], specifically double number lines and tape diagrams to think through problems and make sense of the quantities given, has allowed me to see implicit relationships that are not necessarily given in the problem and has deepened my understanding of the problems. They are now my preferred method of solving fraction, ratio, and proportion problems instead of going directly to an algorithm or algebra. Making sense of the problems visually using VRs has changed the type of math thinker and problem solver I am. I believe using VRs to solve problems cultivates a use of both the right and left side of my brain. I am thinking more creatively and visually about math than I have ever before. The best thing about all of this happening to me, is that I am able to cultivate this same use of VRs with my students and they have begin using VRs as thinking and reasoning tools."
"...my experience with the fix a mix task stood out for my learning [during an online session] because it forced me to use a visual (tape diagram), that I have very little experience with (outside of this course). It put me in an uncomfortable position and forced me to think outside of my algebraic box. It surprised me that I actually liked doing it this way and even shared the problem and my experience with my students. I was pleasantly surprised that I had underestimated their abilities and they jumped at the opportunity to solve the fix a mix task and had several different ways of showing their thinking (they had never had any experience with things like this task before)." [Note the fix-a-mix task linked above is based on the Nana's Paint Mixup Three-Act task by Dan Meyer and includes his video as an adaptation of the acting out with realia strategy; Dan Meyer has many other Three-Act mathematics tasks; note also there are mixing paint VAM apps that can be used with this problem or with related content: Mixing Paint, Comparing Mixtures (12 cups), Comparing Mixtures (24 cups), and Using Ratios to Mix Paints]
Denise Schultz
William Zahner
Peter, thanks for both this video and the amazing resources you are providing in your comments. I really value the work that you and your team at EDC are doing. In fact, the Driscoll Nikula de Piper book is on my desk right now as I am planning for PD efforts for my project (in a shameless plug, my video is here)!
I want to second Beth's and Kathe's questions above. I think you could do an incredible service to the field by making these materials available, either by running your PD using a different model or by publishing the course.
Again, wonderful work and I am amazed by the resources your team is sharing. Thanks!
Peter Tierney-Fife
Curriculum/Instructional Design Associate
Thank you, Bill, and I appreciate your shameless plug. I am excited by your work on conceptually-focused mathematical discussions and appreciative of your support and feedback for the VAM project. The book you mention, Mathematical Thinking and Communication: Access for English Learners, is a resource we use in the VAM course and many educators report it has been helpful, including the sections on differentiating teacher questions and the design principles for mathematics tasks.
We are excited by the feedback we have received about the VAM course, and by the preliminary analyses of our research on the cluster randomized trial. We are actively looking into ways we could offer the VAM course—or a portion or a version of the course!—beyond this funding, as well as ways to make the material available more broadly. Suggestions related to what aspects of the project would be most valuable to different audiences are appreciated as we prioritize our efforts during this phase of our work.
Sarah Sword
Peter, have any of the teachers you work with expanded on what they mean by "the big picture of mathematics" - what it is to them, and (specifically) how it changes their teaching?
Peter Tierney-Fife
Curriculum/Instructional Design Associate
Sarah,
We didn't ask course educators specifically about how participation in the VAM course may have influenced their views of mathematics overall or in a broad sense, but in notebook responses, informal conversations, focus groups, and interviews, educators did sometimes talk about how they felt their participation in the VAM course influenced their thinking on various topics. We are currently working to analyze and code artifacts from the educators who participated in the cluster randomized trial, and we expect to have more to report in the future!
However, in the spirit of trying to not dodge your question entirely, I can write that based on my own engagement with VAM course participants, the type of participant comments I thought of when reading your question relate to feeling a greater understanding of the connections between grades 6 to 8 ratio and proportional relationship representations and concepts—and not necessarily of mathematics more broadly. Some individuals commented in general terms about how they felt this greater understanding of connections among representations and concepts changed their teaching.
Here are some related, short example responses from prior VAM course participants—please keep in mind these were just responses I could find quickly and they are not representative of all participants:
"I think that the course has helped me see more of a common thread throughout those [ratio and proportion] topics."
"Being an 'algebra brain,' I've always struggled with seeing the math that I am being asked to both learn and teach. Through these visuals (double number lines, tape diagrams, etc.) I have been able to better understand the connections and the reasons behind the math that I was taught years ago. It both helps me understand more and be able to explain the relationships in the math (ratios, rates, percents) to my students."
"Once again, I am struck by the relationships.... the work on the coordinate plane shows that at any given point, the coordinates [of a line representing a proportional relationship] can be reduced to the same unit rate. This is also the slope.... Each new strategy is giving me ideas for teaching new concepts to the kids which before I was teaching somewhat in isolation. I can now pull everything together under the idea of "proportions". And each time I introduce a 'new' unit (percent, transformations and dilations, or slope, etc.) I can show them that they already have strategies for understanding the material."
"I have a deeper conceptual understanding of specifically sixth grade math content…. Just to have formal explicit instruction and a reason to focus and emphasize them in my own instruction with students helped grow my personal math conceptual understanding, and… therefore my instruction and the learning of my students."
"When I taught slope to students, I didn't teach it with the current understanding of its connection to proportional relationships and similar triangles. This course has added a lot to my understanding of the connections between these representations."
"In this session, my experience with the handout ‘DNLs, Linear Relationships, and Slope’ [this is a reading we created for the VAM course] added to my own knowledge of linear relationships…. In the past when teaching this to my students I would struggle with being clear when trying to reinforce the concept that all linear relationships are not always proportional."
"At first, I thought that this [online] session was not as useful for my learning as other sessions have been because the fix-a-photo tasks seemed more geared toward 7th and 8th grade standards, but then I realized how useful it was to see the progression and see how what we teach in 6th grade is connected to what is taught in the later grades."
"I'm pleased to finally see how this all leads up to 8th grade standards....I am finally seeing the bigger picture." [note the ellipses were in the original written response; this specific response was in the video; my interpretation from the larger context is that this relates to connections between grades 6 to 8 standards]
"The double number line's versatility has completely taken me by surprise and helped me see the display of content and connections between content in ways that I had never thought of before."
I hope these example responses are helpful.
Sarah Sword
Thanks, Peter - these are really interesting. The teachers really were specific about new connections they made - cool.
Peter Tierney-Fife
Curriculum/Instructional Design Associate
Sarah,
I think one of the nicest things is the delight and, at times, humor some VAM course participants express when writing about shifts in their own thinking and/or teaching practice. I find it very inspiring. For example:
"This course has shown me the importance of the world beyond the algorithm! I have come to see just how limiting the algorithm method is in solving many math tasks and that using VRs [visual representations] effectively expands student possibility for entry into the problem and provides much opportunity for extended thinking. At the start of the course I was hesitant at using VRs. I had not had a lot of practice using them myself so I would tend to avoid making VRs part of my lessons if possible. That has changed for me."
"This has been a big brain shift for me...my math mind has been equation centered for quite some time. I have learned that by asking my students to use VRs, mathematical language production and sharing becomes the focal point of most lessons."
"Before this course, I was relied on finding the algebraic form of any task.... As I began to shift my thinking, I began to observe how my teaching began to shift.... I noticed this change several weeks ago. We were working on our algebra unit when we encountered a problem. I read the problem and I solved it using a visual representation. I took a step back and surprised myself that I did not quickly solve it using my algebraic thinking. In that moment, I was very proud of myself... It was a very important teachable moment because we are not always going to remember rules/formulas but if we understand the problem and use a visual to help us we can then make the connections."
Peter Tierney-Fife
Curriculum/Instructional Design Associate
Thank you to everyone who has watched our video, and thank you particularly to people who have commented in this discussion and subscribed to it. I also want to write a special thank you to all of the VAM course participating educators with whom we have learned so much about mathematics professional development and improving learning and teaching for grades 6 to 8 students who are English learners.
In that spirit, I'll let a couple VAM educators close the discussion:
"This course has forced me to give the students more challenging mathematics than I have in the past and it has been wonderful.... Challenging them with grade level work but giving them the scaffolding or supports they need has pushed them to work harder on their basic skill deficits. Using the visuals helps them to process the information. I have never used drawing visuals as much as I have this year; it is part of our process now. They can see that they can be successful in math and [they] want to work harder."
"I have learned that there are so many simple ways to help ELL students - pictures, diagrams, matching activities, co-created word banks, etc. and that what it takes is some amount of planning... When this year started and I had so many ELL students, I remember feeling worried that I would not be able to help them. But with the help of this [VAM] class, I feel so much better prepared to help and have found ways that have really helped me to connect with these students. I see them using these strategies and I am so excited for their progress and thankful for these additional ideas."
Please contact us at vam@edc.org for more information.
Further posting is closed as the event has ended.